Abstract
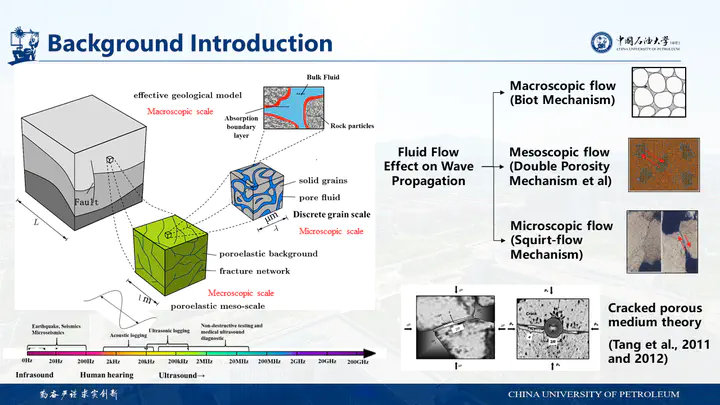
It is universal that rocks contain pores and cracks in the earth’s crust. Multipole acoustic logging in a homogenous fluid-saturated cracked porous formation can be simulated by the realaxis integration (RAI) method based on the cracked porous medium elastic wave theory. However, the analytical solution is invalid to simulate wave-field of the borehole surrounded by inhomogeneous permeable formations which contain both Biot and squirt-flow mechanisms. To solve this problem, we developed a finite-difference time-domain (FDTD) method which extends existing FDTD methods to include an important squirt-flow wave attenuation mechanism and the multipole source excitation. All the complex and frequency-dependent modulus in poro-crack elastic wave equations can be exactly expressed by the Zener mechanical model. Convolutions in the time-domain equations are avoided by introducing memory variables. The non-splitting perfectly matched layer (NPML) is well applied to this poro-crack problem. The proposed FDTD algorithm’s accuracy is validated by the RAI method through comparing simulated multipole acoustic logs in a homogenous cracked porous formation with different crack densities. Finally, acoustic logs in a horizontally stratified formation where a cracked porous layer is sandwiched by a tight porous formation are simulated by the developed finite-difference scheme, providing a theoretical foundation for the borehole acoustic simulation in inhomogeneous permeable formations.
Feiyue Xia(夏飞月)
Ph.D. Student of Petroleum Engineering
Ph.D. Student at UT Austin. My research interests are borehole geophysics, rock physics and petrophysics.